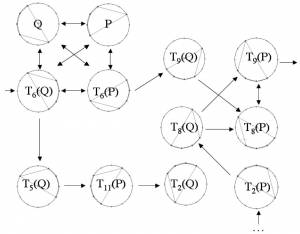
Formalismes Catégoriels en Analyse Musicale

En poursuivant une recherche ouverte il y a une trentaine d'années par Guerino Mazzola dans le domaine de l'applications d'outils issus de la théorie des catégories et des topoï en musique, je m'intéresse aux rapports entre approches transformationnelles en analyse musicale et modélisations catégorielles en musique et en sciences cognitives. Ce domaine de recherche, qui fait l'objet actuellement d'une thèse de doctorat de John Mandereau (en cotutelle UPMC/université de Pise), a également des intersections avec l'axe de recherche autour de l'Analyse Formelle des Concepts et des Structures Conceptuelles appliquées à la musique.

Cette recherche est menée en collaboration avec de mathématiciens et musicologues computationnels (en particulier Guerino Mazzola, Thomas Noll, Andrée Ehresmann, René Guitart et Alexandre Popoff).

Bibliographie

C. Ehresmann "Gattungen von lokalen Strukturen", J. der Deutschen Math.-Vereinigung, Volume: 60, page 49-77, 1957 (disponible en ligne sur EuDLM)

G.S. Halford et W.H. Wilson, "A Category-Theory approach to cognitive development", Cognitive Psychology 12, 356–411, 1980.

G. Mazzola, Gruppen und Kategorien in der Musik: Entwurf einer mathematischen Musiktheorie, Heldermann, 1985

D. Lewin, Generalized Musical Intervals and Transformations, New Haven, Yale University Press, 1987 (new revised edition, Oxford University Press, 2006)

G. Mazzola, M. Andreatta, "From a categorical point of view : K-nets as limit denotators", Perspectives of New Music, vol. 44, n° 2, Août, 2006, p. 88-113

P. Hitzler, M. Krötzsch, G.-Q. Zhang, “A Categorical View on Algebraic Lattices in Formal Concept Analysis.” In Fundamenta Informaticae 74 (2–3), IOS Press, 2006.
Formal concept analysis has grown from a new branch of the mathematical field of lattice theory to a widely recognized tool in Computer Science and elsewhere. In order to fully benefit from this theory, we believe that it can be enriched with notions such as approximation by computation or representability. The latter are commonly studied in denotational semantics and domain theory and captured most prominently by the notion of algebraicity, e.g. of lattices. In this paper, we explore the notion of algebraicity in formal concept analysis from a category-theoretical perspective. To this end, we build on the the notion of approximable concept with a suitable category and show that the latter is equivalent to the category of algebraic lattices. At the same time, the paper provides a relatively comprehensive account of the representation theory of algebraic lattices in the framework of Stone duality, relating well-known structures such as Scott information systems with further formalisms from logic, topology, domains and lattice theory.

M. J. Healy, Th. P. Caudell, “Ontologies and Worlds in Category Theory: Implications for Neural Systems,” Axiomathes 2006 03/06, 16(1-2), 165-214
We propose category theory, the mathematical theory of structure, as a vehicle for defining ontologies in an unambiguous language with analytical and constructive features. Specifically, we apply categorical logic and model theory, based upon viewing an ontology as a sub-category of a category of theories expressed in a formal logic. In addition to providing mathematical rigor, this approach has several advantages. It allows the incremental analysis of ontologies by basing them in an interconnected hierarchy of theories, with an operation on the hierarchy that expresses the formation of complex theories from simple theories that express first principles. Another operation forms abstractions expressing the shared concepts in an array of theories. The use of categorical model theory makes possible the incremental analysis of possible worlds, or instances, for the theories, and the mapping of instances of a theory to instances of its more abstract parts. We describe the theoretical approach by applying it to the semantics of neural networks.

G. Mazzola, La vérité du beau dans la musique (en collaboration avec Y.-K. Ahn), Collection Musique/Sciences, Ircam-Delatour France, 2007

A. Ehresmann, J.-P. Vanbremeersch, Memory Evolutive Systems: Hierarchy, Emergence, Cognition. Elsevier, Amsterdam, 2007. Voir également le cycle de séances spéciales autour des systèmes évolutifs à mémoire (2011-2012) avec la participation de Andrée C. Ehresmann (dans le cadre du séminaire MaMuX).

M. Andreatta, "Calcul algébrique et calcul catégoriel en musique : aspects théoriques et informatiques", Le calcul de la musique, L. Pottier (éd.), Publications de l'université de Saint-Etienne, 2008, p. 429-477

J. Th. Winkler, Algebraische Modellierung von Tonsystemen. Musiktheorie mit mathematischen Mitteln, Beiträge zur Begrifflichen Wissensverarbeitung, Verlag Allgemaine Wissenschaft, Mühltal, 2009

M. J. Healy, “Category Theory as a Mathematics for Formalizing Ontologies,” Theory and Applications of Ontology: Computer Applications 2010, 487-510
Category theory is discussed as an appropriate mathematical basis for the formalization and study of ontologies. It is based upon the notion of the structure manifest in systems of compositional relations and through mappings between systems that preserve composition. With one or two important exceptions, the basic concepts of category theory needed for an understanding of the other chapters involving category theory are presented here. The exceptions will be presented where they are used.

S. Phillips, et W.H. Wilson, Categorical Compositionality: A Category Theory Ex- planation for the Systematicity of Human Cognition. PLoS Computational Biology 6(7), 1–14, 2010

E. Acotto et M. Andreatta, "Between Mind and Mathematics. Different Kinds of Computational Representations of Music", Mathematics and Social Sciences n° 199, p. 9-26, 2012. Special Issue devoted to the Conference of the EMPG 2011 (European Mathematical Psychology Group, Telecom ParisTech, 29-31 août 2011) (pdf)

A. Popoff, Towards A Categorical Approach of Transformational Music Theory preprint pdf

A. Popoff, Building Generalized Neo-Riemannian Groups of Musical Transformations as Extensions preprint pdf

A. Popoff, Using Monoidal Categories in the Transformational Study of Musical Time-Spans and Rhythms preprint pdf

Th. M. Fiore , Th. Noll & R. Satyendra, "Morphisms of generalized interval systems and PR-groups", Journal of Mathematics and Music: Mathematical and Computational Approaches to Music Theory, Analysis, Composition and Performance, 7:1, 3-27, 2013 link

M. Andreatta, A. Ehresmann, R. Guitart, G. Mazzola, "Towards a categorical theory of creativity", Fourth International Conference, MCM 2013, McGill University, Montreal, June 12-14, 2013. Lecture Notes in Computer Science / LNAI, Springer, p. 19-37.
Category theory is discussed as an appropriate mathematical basis for the formalization and study of ontologies. It is based upon the notion of the structure manifest in systems of compositional relations and through mappings between systems that preserve composition. With one or two important exceptions, the basic concepts of category theory needed for an understanding of the other chapters involving category theory are presented here. The exceptions will be presented where they are used.

A. Popoff, An introduction to neo-Riemannian theory (un blog d'initiation aux techniques tranformationnelles en analyse musicale)